The diminishing angle of the ecliptic

deutsch  english   español    français

 

Berlin · 2018  Uwe Topper

The diminishing angle of the ecliptic

Part 1
The new discovery

Two years ago, in 2016, my latest book appeared: "The Solar Year Cross (Das Jahrkreuz)
Jolts and gaps in historical chronology."
There were friendly and affirmative answers, hardly some harsh criticism, as if only well-known or obvious results had been expressed in this book.
Of course, some statements have to be re-examined, pondered or discussed. The precession jolts on which the book focuses more than my previous books need to be understood before they make their way.
On one important point, I deviate from all previous opinions: I reject the alleged strong change in the obliquity of the earth's orbit when the precession pole is displaced.
The obliquity of the earth as regards its orbit, the angle epsilon (ε), is steadily decreasing. Whether this movement is uniform for a long time can only be presumed, I say (on p. 126). In any case, I reject the daring speculations of all predecessors that a "pole shift" drastically changed the earth's obliquity. Earlier, authors tended to refer to the mythological model (such as given by Anaxagoras and even Herodotus): In ancient times, the earth's axis was perpendicular to its orbit, there were no seasons, eternal spring prevailed in our latitudes. I had taken over this view in 1977 ("The Heritage of the Giants"). However, in the section "Objection" (Jahrkreuz 2016, p. 266) I make it clear that I consider this idea to be wrong as far as the last pole shifts are concerned, against all catastrophists of the past centuries as well as those today.
Christoph Marx has throughout his life propulsed the thesis, which is often represented by catastrophists, that before the last jolt the obliquity was about 32° against about 23°30' afterwards (as it is today). He concluded this from the length of the great precession year, which was 36,000 years for Hipparch and is now fixed at 25,900 years.
This is a mistake, I say in my book "Jahrkreuz" (p. 268):
"The obliquity of the ecliptic as stated by Ptolemy was 23.9° or about 24° . If you transfer this angle to the Ptolemaic precession circle of 36,000 years, then there is only one conclusion to be drawn: The annual circulation of the earth (or in the ancient view: the sun) must have taken about five minutes longer than now. It was exactly this value which Hipparch had calculated knowing the shift of the spring equinox in the calendar (with 365 ¼ days): one day in 300 years, which Ptolemy had confirmed."
The assumption of large jumps of the obliquity of the ecliptic in historical time is therefore wrong. For further proof, I looked for the Arabic-writing astronomers like Al-Battani, who had measured the tropical year that was two minutes shorter than ours now, while the angle of the ecliptic decreased slightly (as usual). Therefore, no direct inter-dependence between the two values ​​(year length and epsilon) can be assumed.
Conclusion: "The precession jolt we have defined does not lead to a sudden large change in the value of the ecliptic, at the most it provokes a slight straightening up of the earth by a few minutes of arc. Generally, Epsilon changes only slightly over the course of centuries, regardless of the precession movement." (2016, p. 268).
This conclusion was based on the traditional astronomical values ​​and is consistent with the current situation (referring to the textbook of Julius Dick). In "Jahrkreuz" I emphasized this discovery several times, finally repeated in the section "Draft" (2016, p. 444).
In the description of precession jolts (2016, p. 147), I assure:
"The position of the Earth's axis to its orbit, the obliquity of the ecliptic, does not change significantly, - minimal fluctuations are to be assumed ..."
Although the tropical year length has sometimes increased or decreased dramatically, the obliquity of the ecliptic has not been affected, but has continued to diminish by about the same degree as usual.
I would now like to explain this point a little more broadly, because the following fallacy still haunts the archaeoastronomers' dating methods:
Since "during the last 6,000 years" (wikipedia) the obliquity of the ecliptic continuously decreased, it is quite easy to determine the age of a building (or an astronomical document), if a precise indication of the then pertaining angle epsilon (ε ) is to be found or deductible from it. You only have to reckon backwards in time by using today's equation of epsilon variation and you get the amount of time that has elapsed since then.

Part 2
The measurement problem, using the example of latitude information in antiquity

Looking at the classical values ​​for the northern latitude of many cities of the inhabited world (oecumene), such as Ptolemy (Geografike, "around 140 AD") or his predecessors, especially Strabo and Pliny, also Hipparch, it can be seen that the data are of varying reliability. The vast majority of values ​​are calculated, not measured by using the sun's shadow or a star position, but calculated according to the day length in relation to the night on summer solstice. For the sake of simplicity the data were given in quarter-hour sequencies, which leads to rounded values. In Ptolemy (Syntaxis) the values ​​appear in steps of 5 minutes of arc.

The few extant observed values for latitude (see note 1) are quite good, only a bit too high by 10 to 20 minutes of arc. It is generally concluded (Peschel 1877, p. 43) that this is due to a systematic error produced by applying the upper edge of the sun's shadow of the gnomon, not the center of the sun, which is 16 minutes of arc lower (see Fig. 1). Atmospheric refraction can only amplify this result by one minute of arc, since it is measured when the sun is high in the sky, while near the horizon the increase would be considerable depending on the prevailing humidity.

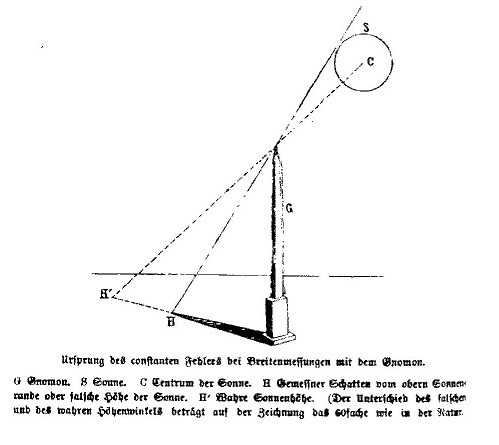
Figure 1 after Peschel p. 44

Against the assertion of the systematic error in applying the edge of the shadow by the ancient astronomers, which is recognized by all recent researchers of history of astronomy, I have an objection: The ancients intendedly set a globe on top of the obelisk and then a small cross or apex above it. Thus the error could be avoided. Also looking through a hole in a standing stone avoids this mistake. Both instruments are extant from antiquity.
The somewhat "too high" epsilon values ​​of the ancient geographers could be justified.
At least this statement stands: Eratosthenes measured a larger angle epsilon than he should have measured when compared to modern calculation; he measured 23° 51' 20". Why?
There are three approaches to answering the question (see also note 2):
1) Why was Eratosthenes wrong?
2) Eratosthenes could not be wrong;
a) his distance from today was greater than historically claimed, or
b) today's projections of the epsilon curve must be wrong.

We regard the displacement of the Earth's axis, the reduction of epsilon, the change of the obliquity of the Earth's equator to its orbit. This decrease of epsilon is measurable in relation to time by means of a modern equation which shows that the latitudes measured by the ancient astronomers are too high.
But if they were reasonable? In that case, the decrease of the earth's obliquity "in past times" would have been faster than we assume today.
Years ago, this answer seemed to me the best, since it does not influence the precession jolts, as I emphasized in 2016: the regular diminishing of the obliquity is too small to have a noticeable effect.
By contrast, the values ​​of megalithic monuments taken seriously by archaeoastronomers make those monuments thousands of years older than estimated hitherto, which I considered unreliable because of the inaccuracy of measuring. At least Peschels statement regarding the edge of the sun is to be considered.

Part 3 : Result

The modern recalculation equation states that the variation in the ecliptic is cyclical over a period of around 40,000 years, i.e. that it returns to a similar value in 40,000 years. It is a descending or ascending curve that takes about 20,000 years half-way. In a graph (Laskar 1986, see note 3) showing the result of the extrapolation of the data measured today and the calculated influence of the planets using the theory of relativity, it is easy to see that the obliquity has been steadily decreasing in the last 8000 years; before that time it was increasing. Around 6000 BC it was at 24° 12', the highest possible point. Around 3000 BC it was at 24°. Since it does not turn abruptly but rather sinusoidally, the increase and decrease at the turning points are very weak. We are now in the middle of the descending curve, which is almost straightforward from 4000 before today (BP) to 3000 after today. It will continue to decrease for a few thousand years, to 22° 30', then gradually increase again.
By conventional assumption, Eratosthenes worked about 2200 years before present. According to the modern equation, he would have had to give an obliquity value of about 23° 43' (about 8' less than the value he measured: 23° 51' 20"). If chronology was shorter (as we assume), Eratosthenes would be brought nearer to us; at a reduction of one millennium he should have measured 23° 36', that is 15' less (a quarter of a degree).
Presumably, the precession jolts will have caused very small fluctuations - mind you: very small. The seasons of the year were not altered by such a slight reduction in the ecliptic by a few minutes of arc, because the difference between 24° 5' (= 24 1/12) (note 4) and 23° 50' is only 15 minutes of arc, which corresponds to the modern calculated difference between today and the Roman Empire. Precessional jolts and similar phenomena of other planets affecting epsilon can account for Eratosthenes' measurements: nature makes leaps, and the ecliptic is no exception to this, but since the jumps are very small they are of ​​no importance to our thesis.

The question was: are today's projections wrong because they presuppose too much regularity of the Earth's motion (and of the solar system in general), or are they wrong, because the amount of bygone years was not properly estimated by historiographers, which means that the correctly projected values pertain to other (earlier) points of the chronology?
In order to reconcile Eratosthenes with the projected values, he would have lived much further away in the past than supposed. A reduction of the chronology (our thesis) would show that the values ​​attributed to Eratosthenes were measured in a very distant past. That would definitely overthrough our view of history.
If we do not want to accept that, then the error is not to be found in the historical chronology but in the assumption of a continuous and uninterrupted movement of the celestial bodies. Already for the time of Copernicus (500 BP) Laskar's curve deviates slightly (it is steeper there). Copernicus has 23° 28', while the equation gives 23° 30' 20", over 2' more. Laskar does not take into account historical documents, but I say with Copernicus (2016, p. 205): When the historically transmitted chaotic changes (jolts etc.) in the course of time are ignored, then a purely theoretical picture of the past, without claim to reality, is the result.

It would be conceivable that epsilon abruptly decreased by a few minutes of arc at a precession jolt and then decreases again by about the current speed. The curve must not have been steeper, it just had to be interrupted. One might even roughly deduce how many minutes of arc epsilon may have changed approximately on each of the last jolts assuming a steady rate corresponding to today's rate (which is an arbitrary but possible assumption).

Literature

Dick, Julius (1965): Grundtatsachen der sphärischen Astronomie (Leipzig)
Mžik, Hans von (1938): Theorie und Grundlagen der darstellenden Erdkunde (Geografike of Ptolemy) with the collaboration of Friedrich Hopfner (Gerold, Vienna)
Peschel, O. (1877): Geschichte der Erdkunde, 2.° (Munich)
Ptolemy - Manitius, Karl (1912-13): The Claudius Ptolemy's Handbook of Astronomy (Teubner, Leipzig, 2 vols, German translation from the Greek, Reprint 1963)
Vitruvius (1908): Ten books on architecture, German trans. and comm. by Franz Reber (Berlin, Wiesbaden 2004)

Notes

Note 1
Peschel 1877, p. 45:
Eratosthenes (in Strabo) has for Alexandria 30° 58' (instead of exactly 31° 11') - 13' higher
Hipparch has for Rhodes 60: 43 5/6 = 36° 8' 7" (instead of 36° 25') - 17' higher
Pitheas has for Marseille 42° 57' (instead of 43° 17' 47") - 20' higher
the town of Canopus is given as 31° 5' (instead of 31° 19' 14") - 14' higher
Peschel wants to show that the ancient values ​​are always around 16' too low, because just the upper sun edge has been accounted for, while the center of the sun at midsummer is lower than the upper edge of the shadow indicates (see his drawing, Peschel p. 44)

Vitruvius, De architectura IX:
Rome 8: 9 = 0.888 = 41° 35' - the exact value today is 41° 53' 30"(18' higher)
Athens 3: 4 = 0.75 = 36° 55'- exactly 37° 59' (64' higher)
Rhodes 5: 7 = 0.714 = 35° 32', - exactly 36° 11' (39' higher)
Tarent 9:11 = 0.82 = 39° 20', - exactly 40° 24' (64' higher)
Alexandria 3: 5 = 0.6 = 31°, - exactly 31° 12' 20" (12' higher)
As the fractions have to be handy (namely geometrically expressible), the results are quite good. All modern values ​​are higher (two even over 1°).
If the latitude (Phi ɸ ) is set too low, (ε) has been higher.

Note 2:
There are also other answers: for example, referring to the drift of the continents; or a drastic shift of the pole (which I reject for the historical period), or random miscalculations.

Note 3 : Laskar 1986 (wikipedia):
"For the past 5 million years, Earth's obliquity has varied between 22° 2′ 33″ and 24° 30′ 16″, with a mean period of 41,040 years. This cycle is a combination of precession and the largest term in the motion of the ecliptic. For the next 1 million years, the cycle will carry the obliquity between 22° 13′ 44″ and 24° 20′ 50″.[NASA]"
wikipedia continues: "The Moon has a stabilizing effect on Earth's obliquity. Frequency map analysis conducted in 1993 suggested that, in the absence of the Moon, the obliquity can change rapidly due to orbital resonances and chaotic behavior of the Solar System, reaching as high as 90° in as little as a few million years."

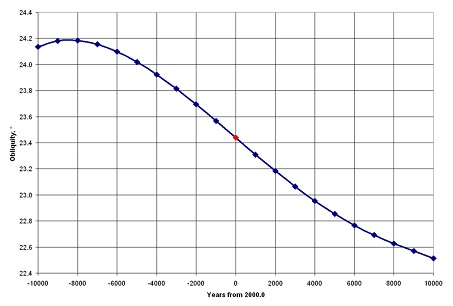
Fig, 2: Algorithm of the change of the obliquity by Laskar 1986

Note 4:
The value of the angle of the obliquity (ε) at the time of Eratosthenes, as he himself is supposed to have calculated, was half of 11/83 of the full circle, that is 23° 51' 20". The latitude of the tropic of cancer (at Syene), used for his calculation of the size of the earth, was just over 24°, exactly 24° 5' (= 24 1/12). The second statement was probably not measured by Eratosthenes himself but taken over from ancient times.
Wikipedia continues: "Already in the 5th century BC, Oynopides of Chios had come to 24° ". This might be realistic because he lived long before Eratosthenes, so epsilon was closer to 24°.

Uwe Topper in cooperation with Ilya Topper – April 6, 2018

I would like to write a comment to this text:

home